For those who prefer French, André Rose has translated this.
What could be easier than stability? Just make the boat wide and it will be stable … right? Yet, there are kayaks out there from 20" to 32" wide, all of which the manufacturers say are stable. After all, what manufacturer is going to say, "you need to be born in a kayak to keep this sucker upright"? How can they all get away with this? And what is "secondary stability" anyway? I know from personal experience that this question will provoke a discussion that can go on for days.
Stability is almost always the first concern of the beginning kayaker. Stability is the first thing an experienced paddler will notice about a kayak, and improper stability performance will immediately disqualify a boat for them. Everything people want to know about the stability of a kayak design is contained in their "stability curve".
Sea Kayaker Magazine has been publishing stability curves with their kayak reviews for some time now. Novices look at the curves and are baffled, assuming that the information must be over their head. Skilled paddlers look at the assumptions involved in creating the curve and feel that they are irrelevant to someone who really knows how to paddle. A little experience can make the curves informative regardless of your paddling skills.
What is Stability
The definition of stability seems pretty clear to most people. A boat that keeps them out of the water is stable, one that dumps them in is not. Although that seems pretty clear cut, two people trying the same boat will still have different opinions about its stability. It is useful to start by agreeing on what it means to be "stable". The dictionary definition that applies to boats in water is probably: "designed so as to develop forces that restore the original condition when disturbed from a condition of equilibrium or steady motion." In a kayak we want to return to an upright position after being "disturbed" by tipping to the side. So a "stable" kayak will develop forces that restore the boat to an upright condition after being leaned or tipped.
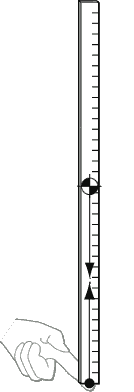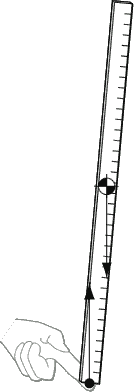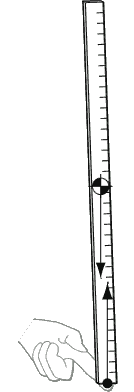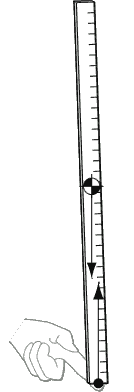
What Forces are Involved
There are two major forces at work on a kayak at rest in the water. The weight of the paddler, his gear and the boat all add up to a force pushing down towards the center of the earth. This weight is supported by an equal and opposite force from the buoyancy of the water, which pushes up. It is the interaction of these two forces that are involved in stability. The relative distribution of the forces will determine whether a kayak is stable or not.
The buoyancy force of the water is distributed over the whole submerged part of the boat. The water pressure pushing on the outer surface of the boat adds together to support all the combined weight in the kayak. Instead of trying to keep track of a bunch of distributed forces engineers generally find a "centroid" or center of force. If you add together all the distributed forces and apply the result through the center of force, this one force would cause the same reaction as all the little forces acting at once. This technique lets a kayak designer combine all the weights in a kayak into a "center of gravity" (CG) or "center of mass" (CM) and all the buoyancy forces into a "center of buoyancy" (CB). Since the force of buoyancy is equal and opposite to the force of gravity, the designer does not even need to pay much attention to what the actual value of the force is. Instead, they can just remember that on flat water the force of gravity is straight down and the force of buoyancy is straight up, and just look at the relative horizontal locations of the CG and CB.
With a boat in equilibrium, the centers of force will be aligned one directly above the other. In a kayak the center of buoyancy will be directly below the center of gravity. This way the buoyancy is pushing straight up towards the weight that pushes straight down.
If some new condition comes along to disrupt the equilibrium, such as wind, a wave or the paddler reaching for an escaped water bottle, the kayak will start to tip. As you tip, your CG moves in the direction you're tipping. Unless the CB moves in response, your weight will be hanging out beyond the buoyancy forces supporting you and you will capsize. In a stable kayak design, the action of tipping the boat rearranges the buoyancy forces to move the CB in the direction of the tilt beyond the CG, thus forcing the kayak upright again. In a stable boat the center of buoyancy moves side to side faster than the center of gravity.
How Stability Works
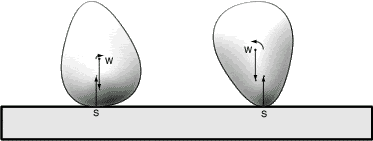
For a kayak to be stable it should either apply a force to push you back to the upright equilibrium condition, or if you want to lean, it should apply force such that the boat finds a new equilibrium condition before it tips you over. The kayak designer controls this by manipulating the cross sectional shape of the kayak and the height of the seat.
Remember that the goal is to keep the CG vertically in line with the CB. Unfortunately, the most stable position is always going to be with the CG hanging below the CB like a rock hanging from a string. But, since you want to breathe, the CG needs to stay directly above the CB. When you move your body to one side, the CG is going to move to that side, away from the CB. To keep you from hanging upside down, the CB now needs to move under you before you rotate all the way over. As the boat rotates in the direction you are tipping, the hull pushes down into the water on that side while the other side lifts out of the water. This action of adding volume (buoyancy) on the side you are tipping and subtracting volume on the other side will cause the center of buoyancy to move toward the side you are tipping. If the boat is shaped to be stable, the CB will move out to the side faster than the CG.
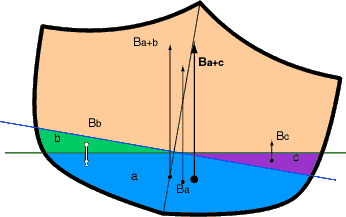
Notice that the change in buoyancy happens due to changes of volume near the waterline. This is why initial stability is dependant on the waterline shape and width and not on the shape below the waterline. Because it is only the water near the waterline that is initially effected by tipping, it is only the shape near the waterline that effects initial stability.
Visualize the end view of a tipping kayak. Picture the narrow slice or wedge of volume being pushed into the water on the side of the tip, and a similar slice lifting out on the other side. As one side is pushed down buoyancy is added on that side and as the other side is lifted buoyancy is subtracted from that side. It is this shifting of the buoyancy which moves the CB. This happens along the whole water plane of the boat. (The water plane is just the outline of the boat at the waterline.) The shift of the buoyancy takes effort. The boat will oppose moving it's CB and it is this tendency of the kayak to resist a change in buoyancy that is felt as the boat's initial stability.
Initial stability is the tendency of the boat to resist tipping a little bit from upright. A larger water plane area increases the volume moving from side-to-side as the boat tips, which requires more effort to move, thus increasing the initial stability. Giving the water plane a greater average width has the same effect.
Notice that the water plane determines the shape and volume of the imaginary wedges and how the CB moves. For small tipping angles, the cross sectional shape of the boat above or below the water plane don't have much effect. This means that the cross sectional shape of the boat does not effect initial stability much. Two kayaks with different cross-section shapes (for example hard chine and soft chine) but similar water plane shapes will have similar initial stability.
It may be contrary to what you have heard people say, but chine shape and whether the bottom is rounded, "V" shaped or flat, will not really effect the initial stability. The shape of the kayak will only effect the stability as it enters or exits the water. While the tipping angle is small parts above or below the water line don't effect the water plane much, so initial stability will not be effected by differences in hull cross sectional shape.
If you are trying two boats with similar water plane shapes and widths and you detect a significant difference in the initial stability it is probably due to difference in seat height or some other factor which changes the height of the CG. The effect of different CG height will be discussed later.
It is only as the tipping angle starts to increase that the cross sectional shape starts to come into play. As the angle increases, parts of the kayak that started above water will enter the water, and parts that used to be wet will become dry.
The center of buoyancy is moved by adding volume on one side (parts getting wet) and subtracting volume on the other side (parts becoming dry). Volume farther away from the original CB will move it faster. One unit of volume 2 inches away is just as effective as two units 1 inch away. The effect of the volume is a "moment" arm dependent on the size of the volume times the distance away. Atlas can move the world with a longer lever because it increases his moment arm.
How much buoyancy force is generated to counteract the tipping force depends on how quickly the CB moves as the kayak tips. Short wide boats create this force by moving a small volume a long way, but narrower boats can create the same effect by moving a larger volume a shorter distance. This righting force or righting moment is often plotted on a stability curve.
Stability Curves
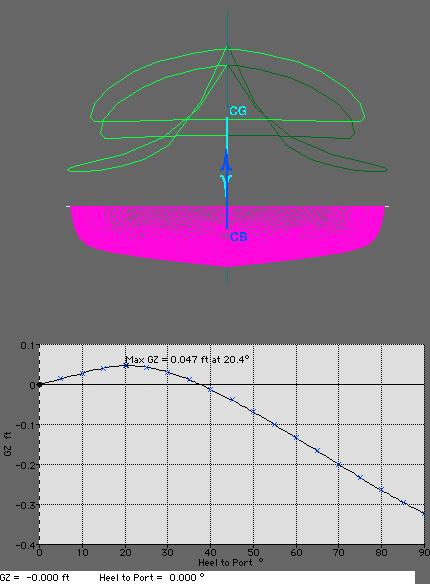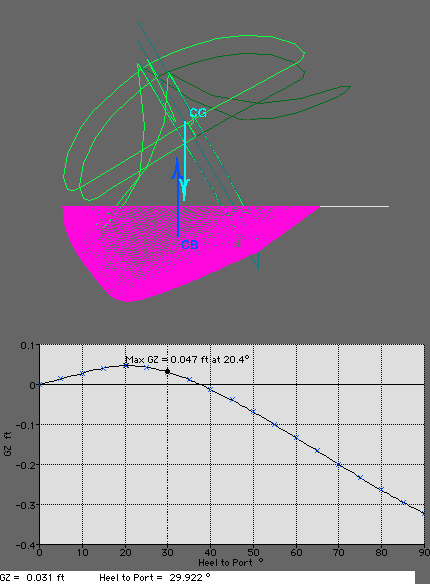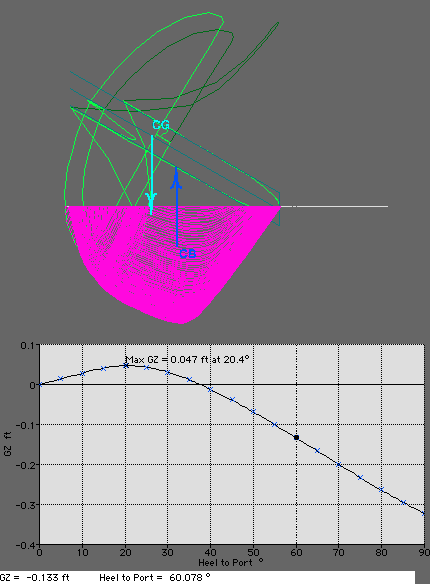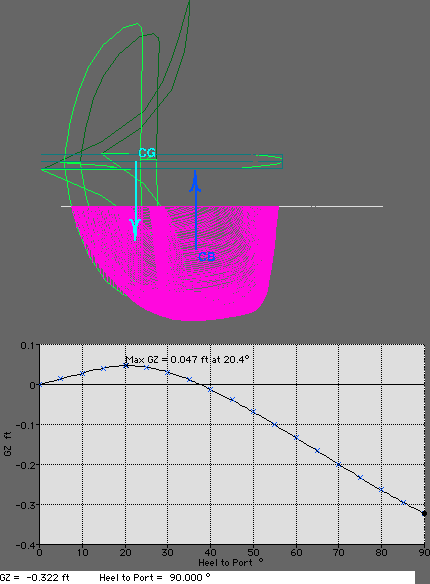
As long as the righting moment is positive, the boat will have a tendancy to return upright unless some other force is applied. When the graph goes negative, you will need to apply some bracing force to return upright.
Often the "Y" axis is given in the units of "foot pounds" instead of "GZ". GZ in this case is in feet. To get "foot pounds" just multiply by the displacement weight in pounds. Feet times pounds equals "foot pounds". So if the weight of the boat is 40 lbs and the paddler weights 200 lbs, maximum will be 0.047 ft x (40+200)lbs = 11.28 foot pounds.
Note: The boat being analyzed above is the Night Heron with a 200 pound load, 10 inches above the bottom of the boat, where the boat weights an additional 37 pounds.
Relative to other performance criteria, the stability characteristics of a boat design are fairly easily quantified. The most common representation of stability is the "stability curve". While units may vary, this graph plots a line proportional to the horizontal distance between the center of gravity and the center of buoyancy for various angles of "heel" or lean. This curve describes the "righting moment", or how much torque the kayak creates to force the boat back upright. It can also be viewed as a "heeling moment" or how much force is required to tip a boat to a given angle. These graphs assume that the paddler remains immobile through out the whole range of angles. That fixes the location of the CG relative to the boat. The designer is left to calculate the location of the CB. This requires some fairly complex integration calculations to determine the center of buoyancy of a series of sections of the boat and then integrating these calculations together to determine the CB for the whole boat. While this is hard to do manually, computers are great at these calculations.
While the rigid paddler may seem silly in a boat like a kayak, which depends on the paddler moving for much of its stability, there are good reasons for this assumption. It eliminates differences due to the paddler's skills and the effect of an active paddler can be deduced from the stability curve.
Reading the Stability Curve
There are several aspects of the stability curve that are worth looking at: the height at a given heel angle, the slope of the curve at any given angle and area under the curve from zero degrees out to a given angle. The height of the curve tells how much force the boat is creating to return upright. The slope of the curve indicates the resistance to further tipping. The area under the curve corresponds to how much energy is absorbed by the boat when it is tipped.
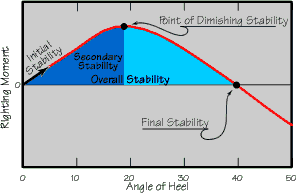
The stability curve (red) can be broken down into several identifiable points. Obviously, the first is the height of the curve. Given any two boats tipped to the same angle, the one with the higher curve at that angle will apply more force to return upright, so it will feel stiffer or more stable. The next thing to look at is the slope of the curve at 0 degrees. The curve that climbs more steeply will have a greater initial stability and feel stiffer. The peak of the curve is where the stability starts to diminish. Having this point either be higher or at a greater angle of heel will make the boat feel like it has more secondary stability. By looking at the area under the curve until the peak of the curve (the dark blue area) you can get one value for the secondary stabilty. The combined blue areas is an indication of how much tipping energy the boat can absorb before a capsize is inevitable. The point of final stability where the line crosses zero is the angle beyond which capsize is inevitable without bracing.
The height of the curve is probably the easiest to understand and is what most people would look at first. A higher curve means the righting moment is greater. This means that it will be harder to tip the boat with the higher value to a given angle and, if two boats are tipped to the same angle, the one with the higher value on the stability curve at that point will start to come upright faster. As long as the stability value is greater than zero the boat will have a tendency to come back upright unless additional tipping forces are applied. So, boats with higher stability curves will generally feel more stable. And boats with positive stability moments out at higher heeling angles will generally give the paddler a little more leeway for tipping. This is easy to understand, but unfortunately is not the end of the story.
Look at the backside of the stability curve where it starts sloping down again and think about what this means. Lets say something hits you with enough force to tip you into this region of the curve and you are lucky and don't tip over. Now another wave comes along and hits you with just slightly more force. You will now be pushed to a place with less ability to push you back. You need more supporting force, and instead you are getting less. Unless you brace you will inevitably go over. Because the curve is sloping downward any increase in tipping will provide a diminishing righting moment. Although the boat still is still providing a righting force, beyond the top of the stability curve this force will not feel very supporting because the slightest additional tipping force will push you down the slope.
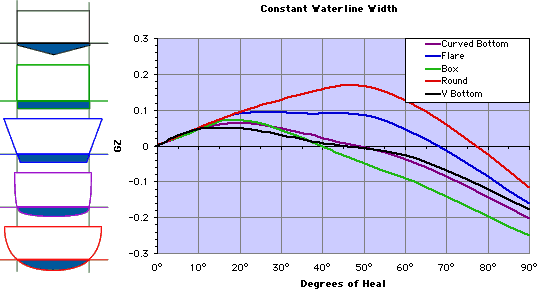
Although you would expect a round bottomed (red) boat to be the least stable, in this case it has the highest overall stability because it flares out a lot above the waterline. And even though the "flared" (blue) shape has similar overall width, the volume distribution of the rounded shape gives it more stability. Any shape that widens above the waterline will tend to have more secondary stability.
Using the same basic hull shapes below are the curves when overall widths are the same. Now, the round bottom is much less stable because the waterline width is much less. This demonstrates why knowing the overall width of a kayak is not that informative. You will learn more by asking for both the overall width plus the width at the waterline.
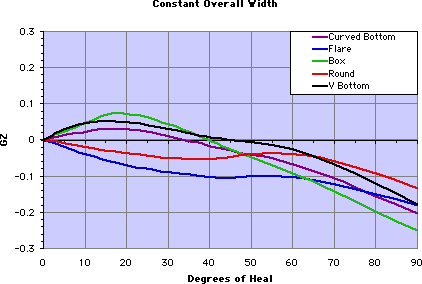
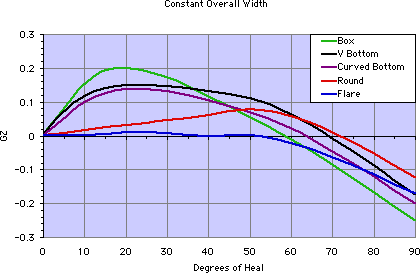
The upward sloping section of the stability curve feels secure because any increase in tipping force will result in a balancing increased righting force. If you want to lean the boat in this section, you can be confident that the boat will support you. A little lack of attention will not generally cause a swim, it will usually just push you back upright.
A rapidly climbing slope has a more solid feel because it takes more force to effect a smaller change. It is like going up a mountain, where a lot of climbing doesn't get you too far on the map. The importance of the slope of the curve is evident at the very beginning. The slope of the curve at small angles of heel corresponds to the initial stability.
As discussed earlier, initial stability is the resistance of the boat to tip just a little bit. The slope of the line at the beginning of the stability curve indicates this resistance. In fact, the slope of the line at any point along the stability curve indicates how much more force will be required to make the kayak tip just a little bit more. Put another way, the slope shows how much an additional tipping force will effect the boat if it is already tipped. A shallower, flatter slope means that an additional force will have more effect.
A subtler difference in feel can be gleaned by looking at how the slope of the line changes as the heel angle increases. If the slope increases at greater angles it means the boat requires a gradually larger addition of force to achieve the same increase in tipping angle. This boat will feel like it stiffens up as you try to lean it over. If the curve has a progressively decreasing slope, the kayak will require a gradually decreasing change in force to create the same change in tip angle. This will make the boat feel like it gets a little looser as you try to lean it over. All boats will have a part of the stability curve which looks like this, where the line is curving downwards like the top of an "n". It will always occur before the maximum point on the curve. The upward curvature of the boat which stiffens will only be found in some designs.
Secondary stability is generally related to the maximum height of the stability curve. Obviously, a higher maximum righting moment will be more stable because you would need to apply more force to reach that angle. But the angle at which the curve reaches the maximum is also important because that indicates how far you can heel the boat before you begin losing stability. One way of combining the height and angle of maximum righting moment is to look at the size of the area between the curve and the horizontal zero line. This indicates the work or energy required in tipping the boat to that point. A larger area under the curve indicates that it will take more effort to tip the boat.
Most people will not feel secure when tipped all the way to the angle where the curve reaches a maximum. The slope of the curve will always level out before going down hill and a horizontal line on the curve means that a small increase in tipping force can create a large change in how much the boat actually tips. And if you are pushed over to where the curve starts sloping downward, you are on the slippery slope where any added tipping force could cause a capsize.
Each person's perception of a kayak's secondary stability will be effected by how comfortable he or she feels when the curve starts to flatten out. There will probably be a point before the top of the stability curve where a little more tipping force causes too much more tip for the paddler's comfort.
Capsize is not inevitable after the high point on the stability curve. The kayak can be leaned all the way over to the angle where the line crosses zero before the kayak will actively help capsizing. This is the point where the righting moment becomes negative and is where the boat is no longer providing any force to push you back towards an upright position. A negative righting moment means the boats buoyancy and your weight are acting to push you farther over.
Again it is useful to look at the area under the curve. Measuring the area below the stability curve from upright out to the angle where the line crosses zero describes the overall stability of the kayak. A larger area under the curve indicates the paddler can be hit with a larger capsizing force and still recover. The area is a measure of how much energy the kayak can absorb without capsizing. The energy may come from leaning you body, a wave slapping into the side of the boat, or a fish pulling on a fishing rod.
Most paddlers will probably not experience the overall stability in regular use. Usually by the time the boat is pushed over the top of the stability curve, the paddler is bracing strongly or taking a deep breath. However, the overall stability will determine how strong a brace is needed or how much time there is to grab that breath.
Interpreting it all.
Just knowing how to read the stability curve is not going to tell a novice paddler what boat to buy. You must first understand how the curve relates to your paddling style and skills. If you can calibrate yourself by trying several boats and reading their stability curves and then being aware of how their stability changes as you lean the boat, you can learn to relate that knowledge to the stability curves of boats you have not tried yet.
Everyone is a different shape or weight. The stability curve assumes a fixed center of gravity for the paddler. For example the reviews in Sea Kayaker assume the CG is 10 inches above the lowest part of the seat. Obviously, many people will not match this assumption. A barrel chested man will have a different CG from a petite woman. Their weight will be different as well as the relative height they carry the weight.
However, just because you do not fit the assumptions does not mean the stability curve is irrelevant. Changing the weight and the height of the CG will have predictable effects on the stability. A different weight paddler will change how deeply the boat sits in the water. This will change the waterline width, and the relative cross sectional shape. However it doesn't really change the shape of the boat and the form of the curve will stay similar regardless of the weight. Lighter people will probably find all boats somewhat more stable than heavier people would find the same boats. Since the boat is floating higher, the length of the right moment arm tends to be longer for lighter paddlers, but since they weigh less the actual righting moment does not change as much as would be expected. As a result the initial and secondary stability often remain surprisingly similar regardless of weight. The overall stability will be less for heavier paddlers. Once they get over the high point on the stability curve, their weight starts to pull them down more quickly.
Raising or lowering the CG will effect the stability in a predictable manner. The exact change in stability can actually be calculated based on how much the CG is changed, but since it the change in stability depends on the paddlers themselves instead of the design of the kayak, nobody needs to do any math. A paddler with a higher center of gravity than his friends will consistently find boats less stable. Shorter paddlers will always find boats more stable.
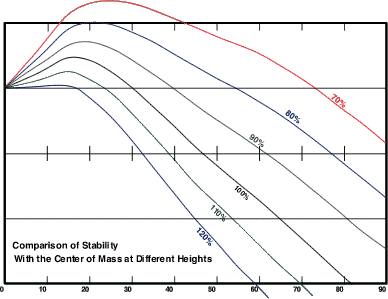
You don't really need to know anything specific about how you differ from the assumptions used in creating the stability curves. If you feel the initial stability is insufficient in one design, any design with the same slope at the beginning of the stability curve will feel equally unstable. This only falls apart if the stability curves you are comparing use different assumptions. That is why it is important that all the reviews published in Sea Kayaker put the CG of the paddler a standard 10 inches above the seat.
Even though most skilled kayakers use leaning and the ability to brace as important parts of their stability when negotiating rough water, the assumption of a rigid paddler used in creating the stability curves is still good. Like moving the CG up and down, changing the CG from side to side produces changes in the stability which are independent of the design of the kayak. Although factors like seat configuration may change how easily the paddler can move around, the ability to lean and edge a boat is highly skill dependant. A boat with a steep stability curve will be hard for skilled paddler to lean up on edge but that will be true of all boats with a similar stability curve. If the paddler has the skill or flexibility to lean a boat, that skill will be able to be translated consistently over a variety of boats. By learning how your skills interact with a variety of different kayaks you can learn to translate the stability curve into something meaningful to you.
Conclusion
Stability is a subjective thing. The same boat that is a threatening death trap for a novice may be stodgy and boring to an experienced extreme paddler. You can never be sure of the personal preferences of someone describing a boat. A salesman eager to sell a boat will tend to emphasize the characteristics he thinks will most appeal to a buyer. The stability curve eliminates this subjectivity. It is the unfiltered reaction of the boat itself.
If you are willing to take a little time to learn how to interpret it, the stability curve can be a useful evaluation tool. While there can be no substitute in the final analysis for spending some quality time in a kayak, learning to relate your on-the-water experience with the stability curve can help narrow down your kayak choices. Even just the awareness of different characteristics on the curve, such as the slope, height and area under the curve, will help you know what to look for as you try out boats.
Try leaning boats as you test them, then refer to old issues of Sea Kayaker to study the stability curves. Then try to identify what you liked and disliked about the stability on the stability curve. At the very least you will burn a little more knowledge about kayak performance into your brain which will serve you well as your skills continue to evolve.